
|
|
|
|
|
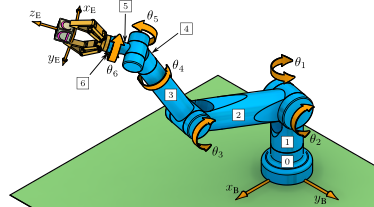
Example 4.08:: Offset Spherical Manipulator: Algebraic Solution (MATLAB)
This example uses the algebraic method to compute the inverse kinematics for the offset spherical manipulator.
Contents
- Clear All Workspace Objects and Reset All Assumptions
- Structural Parameters
- Position and Orientation for OXYZ6
- Normal, Orientation, Approach, and Position Vectors
- Right-Shoulder Solution for

- Solution for
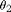
- Solution for
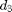
- Solution for
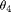 , No-Flip
, No-Flip - Solution for
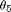 , No-Flip
, No-Flip - Solution for
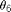 , No-Flip
, No-Flip - Solution for
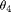 , Flip
, Flip - Solution for
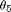 , Flip
, Flip - Solution for
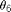 , Flip
, Flip
Clear All Workspace Objects and Reset All Assumptions
clear all
Structural Parameters
d2 = 18; %cm
Position and Orientation for OXYZ6
Given information.
T6N = [ 0 0 1 18
0 1 0 50
-1 0 0 0
0 0 0 1 ];
Normal, Orientation, Approach, and Position Vectors
Extract these vectors from T6N.
nx = T6N(1,1); ny = T6N(2,1); nz = T6N(3,1); ox = T6N(1,2); oy = T6N(2,2); oz = T6N(3,2); ax = T6N(1,3); ay = T6N(2,3); az = T6N(3,3); px = T6N(1,4); py = T6N(2,4); pz = T6N(3,4);
Right-Shoulder Solution for 
theta1R = atan2d(py, px) + atan2d(d2, sqrt(px*px + py*py - d2*d2)); % deg theta1 = theta1R % deg
theta1 =
90
Solution for 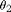
theta2 = atan2d(-pz*cosd(theta1) - py*sind(theta1), pz) % deg
theta2 = -90
Solution for 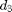
V114 = px*cosd(theta1) + py*sind(theta1);
d3 = -V114*sind(theta2) + pz*cosd(theta2) % cm
d3 =
50
Solution for 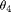 , No-Flip
, No-Flip
V113 = ax*cosd(theta1) + ay*sind(theta1);
V133 = ax*sind(theta1) - ay*cosd(theta1);
V213 = az*sind(theta2) + V113*cosd(theta2);
theta4NF = atan2d(V133, -V213);
theta4 = theta4NF % deg
theta4 =
90
Solution for 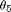 , No-Flip
, No-Flip
V233 = az*cosd(theta2) - V113*sind(theta2);
theta5NF = atan2d(V133*sind(theta4) - V213*cosd(theta4), V233);
theta5 = theta5NF % deg
theta5 =
90
Solution for 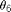 , No-Flip
, No-Flip
V112 = ox*cosd(theta1) + oy*sind(theta1);
V132 = ox*sind(theta1) - oy*cosd(theta1);
V212 = oz*sind(theta2) + V112*cosd(theta2);
V232 = oz*cosd(theta2) - V112*sind(theta2);
V412 = V212*cosd(theta4) - V132*sind(theta4);
V432 = V212*sind(theta4) + V132*cosd(theta4);
theta6NF = atan2d(-V232*sind(theta5) - V412*cosd(theta5), - V432);
theta6 = theta6NF % deg
theta6 = -90
Solution for 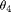 , Flip
, Flip
theta4F = atan2d(-V133, V213);
theta4 = theta4F % deg
theta4 = -90
Solution for 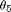 , Flip
, Flip
theta5F = atan2d(V133*sind(theta4) - V213*cosd(theta4), V233); % deg theta5 = theta5F % deg
theta5 = -90
Solution for 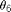 , Flip
, Flip
V412 = V212*cosd(theta4) - V132*sind(theta4); V432 = V212*sind(theta4) + V132*cosd(theta4); theta6F = atan2d(-V232*sind(theta5) - V412*cosd(theta5), - V432); % deg theta6 = theta6F % deg
theta6 =
90
This MATLAB example illustrates a computation from the textbook Fundamentals of Robot Mechanics by G. L. Long, Quintus-Hyperion Press, 2015. See http://www.RobotMechanicsControl.info for additional relevant files.